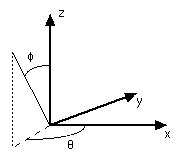
Spherical coordinates are more convenient than Cartesian coordinates when describing an atom.
If spherical coordinates are used, the equations that describe the electron distribution around the nucleus can be separated into two parts, a radial distribution function and an angular distribution function.